Penrose

Penrose tilings are non-periodic tilings of the plane discovered by mathematician Roger Penrose in the 1970s. These tilings bridge the gap between crystallographic patterns and completely random arrangements, displaying what we call “quasiperiodicity.”

The de Bruijn pentagrid method, developed by Nicolaas Govert de Bruijn in 1981, provides an elegant way to construct Penrose tilings. Here’s how it works:

1. Start with five families of parallel lines, where each family is rotated by 72° (π/5 radians) relative to the next. Each line in a family is spaced at regular intervals.
2. Each line in the grid is given a special number (called its “index”). The intersection points of these lines, when following certain rules about their indices, determine the vertices of the Penrose tiles.
3. Lastly, move those tiles around so that their edges are aligned. You can try dragging some rhombuses to form a Penrose tiling here!

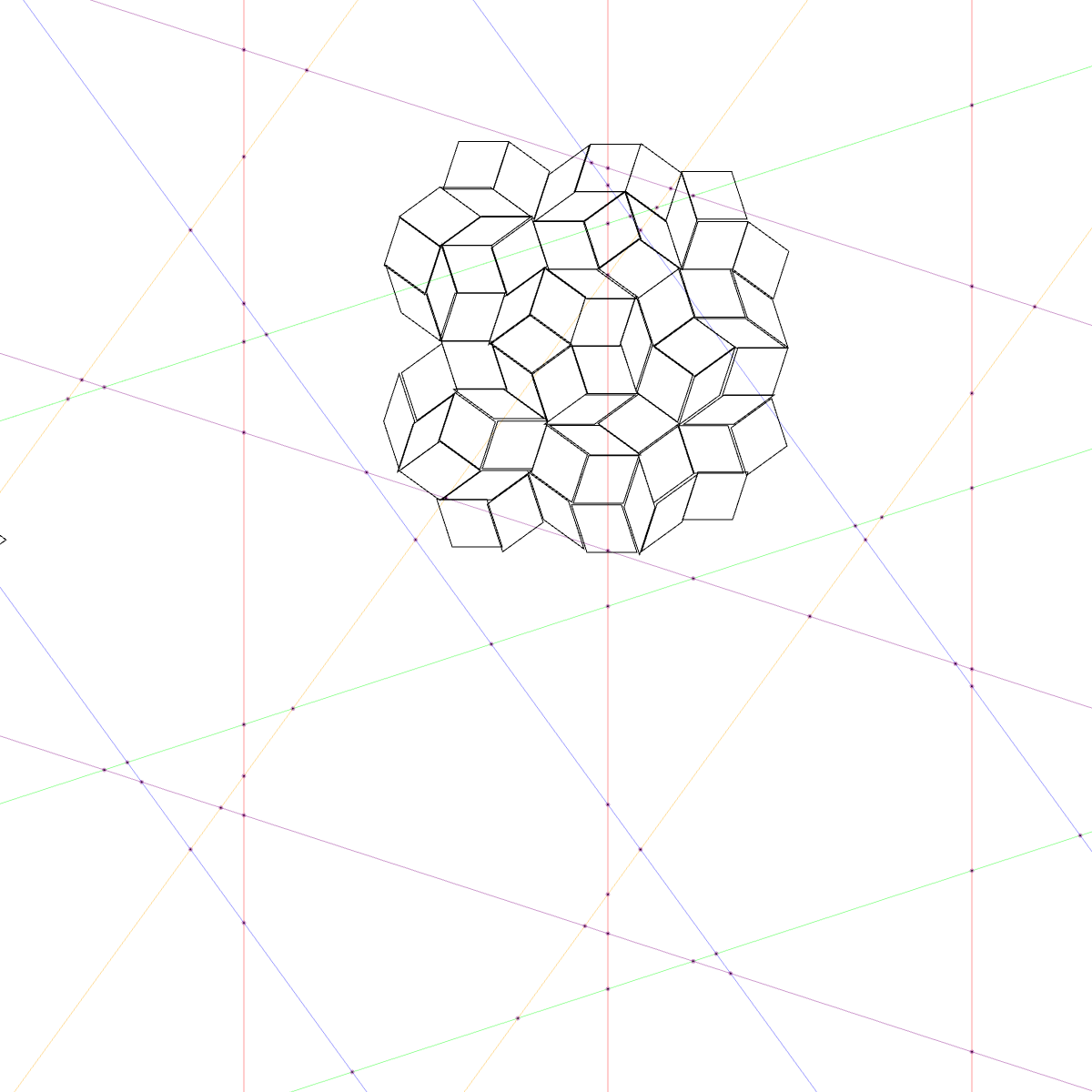
This is also a very cool visualization of Penrose tilings.